Welcome to the best Watts Volts Amps Ohms Calculator, a powerful tool for electrical calculations! Whether you’re an engineer, electrician, or hobbyist, this user-friendly calculator enables you to quickly find Watts, Volts, Amps, and Ohms in electrical circuits. Simply input any two known values, and our calculator will effortlessly compute the missing two quantities using Ohm’s Law. Simplify your electrical tasks and design processes with our comprehensive Watts Volts Amps Ohms Calculator!
| Resistance (R): | ||
| Current (I): | ||
| Voltage (V): | ||
| Power (P): | ||
What is a Watts Volts Amps Ohms Calculator?
A Watts Volts Amps Ohms calculator is a tool used to perform calculations related to electrical quantities in electrical circuits. It is based on Ohm’s law, which describes the relationship between voltage (V), current (I), and resistance (R) in an electrical circuit. The calculator allows users to input known values of two electrical quantities and automatically calculates the other two values using the formulas derived from Ohm’s law.
Here’s a brief explanation of each of the electrical quantities involved:
- Watts (W): Watts are the unit of power, representing the rate at which work is done, or energy is transferred in an electrical circuit.
- Volts (V): Volts are the unit of voltage which measures the electrical potential difference between two points in a circuit.
- Amps (A): Amps are the unit of current, representing the flow of electrical charge through a conductor.
- Ohms (Ω): Ohms are the unit of electrical resistance, measuring the opposition to the flow of current in a circuit.
The calculator allows users to input values in any two of these quantities, and based on that input, it calculates the missing values using the following equations derived from Ohm’s law:
Ohm’s Law Formulas:
- Voltage (V) = Current (I) * Resistance (R)
- Current (I) = Voltage (V) / Resistance (R)
- Resistance (R) = Voltage (V) / Current (I)
- Power (W) = Voltage (V) * Current (I)
Using these formulas, the calculator helps users quickly determine the unknown electrical quantity in an electrical circuit based on the known values.
For example, if you know the resistance and voltage in a circuit, you can use the calculator to find the current and power. Similarly, if you know the current and resistance, you can use the calculator to find the voltage and power. This tool is widely used by engineers, electricians, and hobbyists for various electrical calculations and circuit design tasks.
How does this Watts Volts Amps Ohms Calculator work?
The Watts Volts Amps Ohms calculator works based on Ohm’s law, a fundamental principle in electrical circuits. Ohm’s law describes the relationship between voltage (V), current (I), and resistance (R) in an electrical circuit:
V = I * R
Where: V = Voltage (in volts) I = Current (in amps) R = Resistance (in ohms)
Process:
The calculator allows users to input known values of any two electrical quantities, and it automatically calculates the missing two values using the formulas derived from Ohm’s law. Here’s how it works step-by-step:
- User Input: The user enters known values for any two of the four electrical quantities: Watts (W), Volts (V), Amps (A), and Ohms (Ω). The user can input these values using the provided input fields in the calculator.
- Calculations: Based on the user’s input, the calculator performs the necessary calculations using Ohm’s law equations to determine the missing two values.
- Display Results: The calculator then displays the calculated values for the missing two electrical quantities in the corresponding input fields. These results are automatically updated as the user enters or changes the known values.
- Reset: The calculator also provides a “Reset” button, allowing the user to clear all the input fields and the calculated results to start over or perform new calculations.
For example, if the user enters the values for resistance (R) and current (I), the calculator will use the formula V = I * R to calculate the voltage (V) and power (W). Similarly, if the user provides values for voltage (V) and resistance (R), the calculator will use the formula I = V / R to calculate the current (I) and power (W). For Power calculation, P = V × I formula is used.
The calculator follows the same principles for all possible combinations of input values, allowing users to quickly determine the unknown electrical quantities in an electrical circuit without manually performing the calculations using pen and paper. It simplifies working with electrical circuits, making it a valuable tool for engineers, electricians, and hobbyists in their daily work and projects.
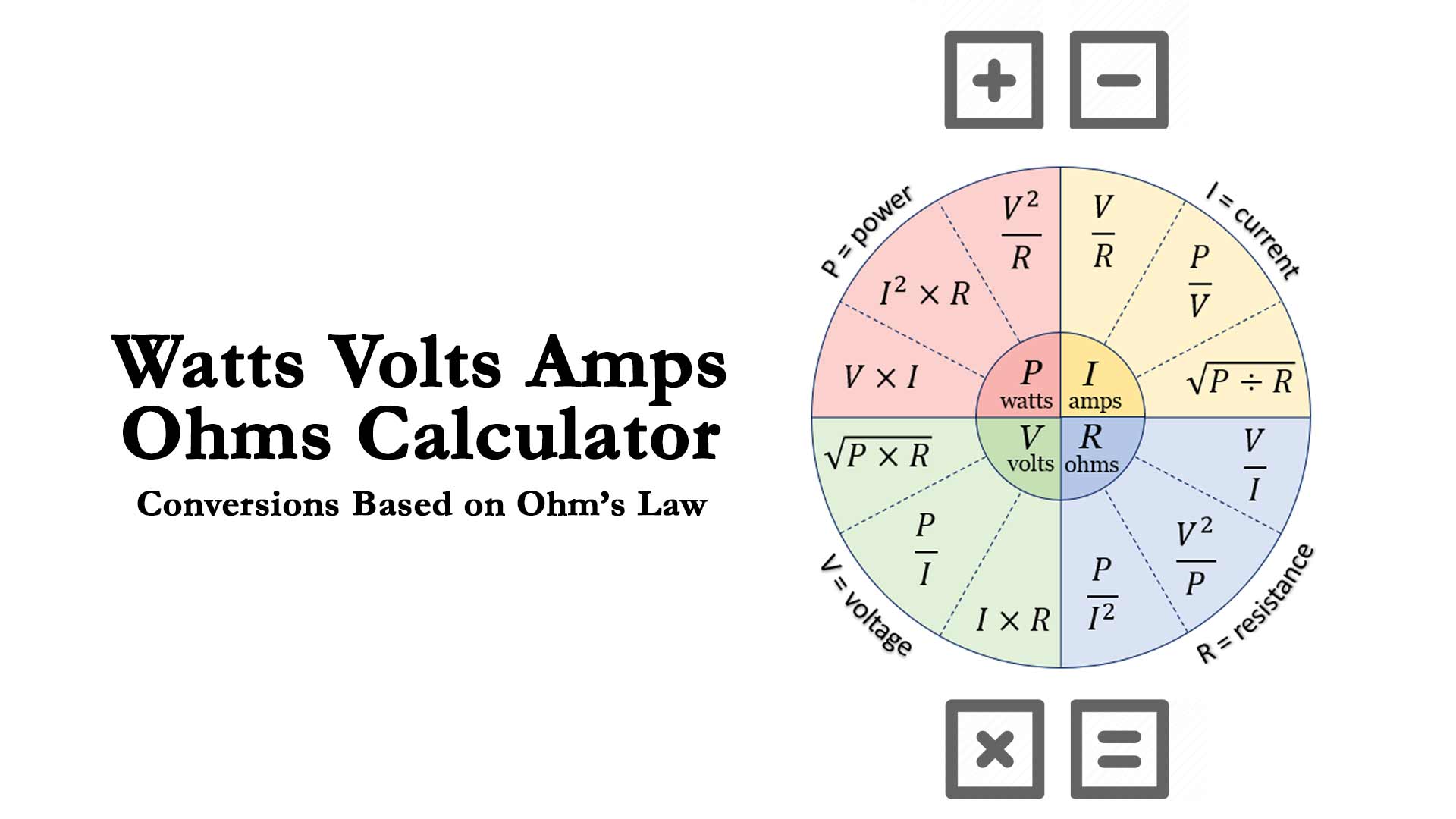
Formulas
Ohm’s Law is a fundamental principle in electrical engineering that describes the relationship between voltage, current, and resistance in an electrical circuit. It is essential for understanding and analyzing various electrical systems, ranging from simple circuits to complex electronic devices. This article explores Ohm’s Law and its associated formulas for calculating electrical circuit resistance, current, voltage, and power. We will delve into the applications of these formulas and highlight their importance in electrical engineering and everyday life.
Ohms Calculations:
- Resistance (R) = Voltage (V) / Current (I): The resistance in an electrical circuit is determined by dividing the voltage (V) in volts by the current (I) in amperes. This formula, R = V / I, provides a direct relationship between voltage, current, and resistance, enabling engineers to measure and control resistance in various circuits.
- Resistance (R) = Voltage (V)^2 / Power (P): Another way to calculate resistance (R) is by squaring the voltage (V) in volts and dividing it by the power (P) in watts. This formula, R = V^2 / P, is valuable in scenarios where voltage and power are known, but resistance needs to be determined.
- Resistance (R) = Power (P) / Current (I)^2: The third formula for calculating resistance involves dividing the power (P) in watts by the square of the current (I) in amperes. The resulting value provides the resistance (R) in ohms. This formula, R = P / I^2, is useful when power and current are given and resistance needs to be derived.
Amps Calculations:
- Current (I) = Voltage (V) / Resistance (R): To find the current (I) in amperes, engineers divide the voltage (V) in volts by the resistance (R) in ohms. This formula, I = V / R, is crucial in determining the current flow through a circuit.
- Current (I) = Power (P) / Voltage (V): Alternatively, the current (I) can be calculated by dividing the power (P) in watts by the voltage (V) in volts. This formula, I = P / V, provides insights into the current when power and voltage are known.
- Current (I) = Square Root of (Power (P) / Resistance (R)): When power (P) and resistance (R) are known, this formula calculates the current (I) in amperes by taking the square root of the power divided by resistance. It is expressed as I = √(P / R).
Volts Calculations:
- Voltage (V) = Current (I) * Resistance (R): Engineers can find the voltage (V) in volts by multiplying the current (I) in amperes by the resistance (R) in ohms. This formula, V = I * R, is widely used to determine voltage in circuits.
- Voltage (V) = Power (P) / Current (I): Alternatively, voltage (V) can be calculated by dividing the power (P) in watts by the current (I) in amperes. This formula, V = P / I, is valuable in understanding voltage levels when power and current are given.
- Voltage (V) = Square Root of (Power (P) * Resistance (R)): When power (P) and resistance (R) are known, this formula calculates the voltage (V) in volts by taking the square root of the product of power and resistance. It is expressed as V = √(P * R).
Watts Calculation:
- Power (P) = Voltage (V) * Current (I): Power (P) in watts can be determined by multiplying the voltage (V) in volts by the current (I) in amperes. This formula, P = V * I, is fundamental for assessing electrical device power consumption or output.
- Power (P) = Voltage (V)^2 / Resistance (R): Another method to calculate power (P) is by squaring the voltage (V) in volts and dividing it by the resistance (R) in ohms. This formula, P = V^2 / R, finds application in scenarios where voltage and resistance are known, but power needs to be calculated.
- Power (P) = Current (I)^2 * Resistance (R): The third formula for calculating power involves squaring the current (I) in amperes and then multiplying it by the resistance (R) in ohms. This formula, P = I^2 * R, is particularly useful in situations where current and resistance are known and power needs to be determined.
The outcome of the formulas:
Ohm’s Law and its associated formulas play a pivotal role in understanding electrical circuits, facilitating voltage, current, resistance, and power calculations. Engineers and electricians rely on these formulas to analyze, design, and troubleshoot electrical systems in a wide range of applications. By utilizing Ohm’s Law, professionals can ensure the efficient and safe operation of electronic devices and electrical networks, making it a fundamental principle in electrical engineering.
How to find amps with volts and ohms?
To find the current (amps) in an electrical circuit when given the voltage (volts) and resistance (ohms), you can use Ohm’s Law. Ohm’s Law states that:
Current (I) = Voltage (V) / Resistance (R)
To find the current (I), follow these steps:
- Identify the known values:
- Voltage (V) in volts
- Resistance (R) in ohms
- Use the formula:
- Current (I) = Voltage (V) / Resistance (R)
- Perform the calculation:
- Divide the voltage (V) by the resistance (R) to get the current (I) in amperes (amps).
Example: Let’s say you have a circuit with a voltage of 12 volts and a resistance of 4 ohms. To find the current in the circuit:
Current (I) = 12 volts / 4 ohms Current (I) = 3 amps
So, the current flowing through the circuit is 3 amperes (amps).
The table on finding amps from volts and ohms
| Example | Volts (V) | Ohms (Ω) | Amps (A) |
|---|---|---|---|
| 1 | 15 | 5 | 3 |
| 2 | 30 | 10 | 3 |
| 3 | 18 | 6 | 3 |
| 4 | 12 | 4 | 3 |
| 5 | 24 | 8 | 3 |
| 6 | 27 | 9 | 3 |
| 7 | 36 | 12 | 3 |
| 8 | 45 | 15 | 3 |
| 9 | 60 | 20 | 3 |
| 10 | 48 | 16 | 3 |
| 11 | 21 | 7 | 3 |
| 12 | 90 | 30 | 3 |
| 13 | 42 | 14 | 3 |
| 14 | 72 | 24 | 3 |
| 15 | 33 | 11 | 3 |
| 16 | 54 | 18 | 3 |
| 17 | 39 | 13 | 3 |
| 18 | 81 | 27 | 3 |
| 19 | 66 | 22 | 3 |
| 20 | 51 | 17 | 3 |
| 21 | 78 | 26 | 3 |
| 22 | 57 | 19 | 3 |
| 23 | 69 | 23 | 3 |
| 24 | 63 | 21 | 3 |
| 25 | 87 | 29 | 3 |
| 26 | 75 | 25 | 3 |
| 27 | 93 | 31 | 3 |
| 28 | 99 | 33 | 3 |
| 29 | 102 | 34 | 3 |
| 30 | 96 | 32 | 3 |
How to find amps with watts and volts?
use the formula:
Current (I) = Power (P) / Voltage (V)
To find the current (I), follow these steps:
- Identify the known values:
- Power (P) in watts
- Voltage (V) in volts
- Use the formula:
- Current (I) = Power (P) / Voltage (V)
- Perform the calculation:
- Divide the power (P) by the voltage (V) to get the current (I) in amperes (amps).
Example: Let’s say you have a circuit with a power consumption of 120 watts and a voltage of 12 volts. To find the current in the circuit:
Current (I) = 120 watts / 12 volts Current (I) = 10 amps
So, the current flowing through the circuit is 10 amperes (amps).
The table on finding amps from watts and volts
Sure, here’s a table showing 30 examples of calculations to find the current (amps) with different values for watts and volts:
| Example | Watts (W) | Volts (V) | Amps (A) |
|---|---|---|---|
| 1 | 50 | 10 | 5 |
| 2 | 120 | 40 | 3 |
| 3 | 80 | 20 | 4 |
| 4 | 240 | 120 | 2 |
| 5 | 100 | 50 | 2 |
| 6 | 360 | 180 | 2 |
| 7 | 150 | 30 | 5 |
| 8 | 480 | 240 | 2 |
| 9 | 200 | 40 | 5 |
| 10 | 600 | 300 | 2 |
| 11 | 250 | 50 | 5 |
| 12 | 720 | 240 | 3 |
| 13 | 300 | 60 | 5 |
| 14 | 840 | 280 | 3 |
| 15 | 400 | 100 | 4 |
| 16 | 960 | 240 | 4 |
| 17 | 500 | 100 | 5 |
| 18 | 1080 | 360 | 3 |
| 19 | 600 | 200 | 3 |
| 20 | 1200 | 240 | 5 |
| 21 | 700 | 140 | 5 |
| 22 | 1440 | 240 | 6 |
| 23 | 800 | 200 | 4 |
| 24 | 1680 | 280 | 6 |
| 25 | 900 | 180 | 5 |
| 26 | 1920 | 240 | 8 |
| 27 | 1000 | 100 | 10 |
| 28 | 2160 | 360 | 6 |
| 29 | 1100 | 110 | 10 |
| 30 | 2400 | 240 | 10 |
How to find watts from volts and amps?
It is really easy and simple to find watts from volts and amps. To find the power (watts) in an electrical circuit when given the voltage (volts) and current (amps), you should use the formula:
Power (P) = Voltage (V) × Current (I)
For finding the power (P), follow these steps:
- Identify the known values:
- Voltage (V) in volts
- Current (I) in amperes
- Use the formula:
- Power (P) = Voltage (V) × Current (I)
- Perform the calculation:
- Multiply the voltage (V) by the current (I) to get the power (P) in watts.
Let me give an example: Let’s say you have a circuit with a voltage of 24 volts and a current of 5 amps. To find the power consumed in the circuit:
Power (P) = 24 volts × 5 amps Power (P) = 120 watts
So, the power consumed in the circuit is 120 watts.
The Table on finding watts from volts and amps
| Example | Volts (V) | Amps (A) | Watts (W) |
|---|---|---|---|
| 1 | 12 | 2 | 24 |
| 2 | 24 | 1 | 24 |
| 3 | 10 | 3 | 30 |
| 4 | 18 | 2 | 36 |
| 5 | 15 | 2 | 30 |
| 6 | 30 | 1 | 30 |
| 7 | 25 | 2 | 50 |
| 8 | 36 | 1 | 36 |
| 9 | 20 | 3 | 60 |
| 10 | 48 | 1 | 48 |
| 11 | 22 | 2 | 44 |
| 12 | 54 | 1 | 54 |
| 13 | 30 | 2 | 60 |
| 14 | 60 | 1 | 60 |
| 15 | 35 | 2 | 70 |
| 16 | 72 | 1 | 72 |
| 17 | 40 | 2 | 80 |
| 18 | 15 | 5 | 75 |
| 19 | 80 | 1 | 80 |
| 20 | 45 | 2 | 90 |
| 21 | 90 | 1 | 90 |
| 22 | 50 | 2 | 100 |
| 23 | 96 | 1 | 96 |
| 24 | 55 | 2 | 110 |
| 25 | 100 | 1 | 100 |
| 26 | 60 | 2 | 120 |
| 27 | 110 | 1 | 110 |
| 28 | 65 | 2 | 130 |
| 29 | 120 | 1 | 120 |
| 30 | 70 | 2 | 140 |
How to find ohms with volts and amps?
In order to detect the resistance (ohms) in an electrical circuit when the voltage (volts) and current (amps) are given, you should use Ohm’s Law.
Here is the Ohm’s Law:
Resistance (R) = Voltage (V) / Current (I)
To find the resistance (R), follow these steps below:
- Identify the known values:
- Voltage (V) in volts
- Current (I) in amperes
- Use the formula:
- Resistance (R) = Voltage (V) / Current (I)
- Perform the calculation:
- Divide the voltage (V) by the current (I) to get the resistance (R) in ohms.
Example: Let’s say you have a circuit with a voltage of 12 volts and a current of 2 amps. To find the resistance of the circuit:
Resistance (R) = 12 volts / 2 amps Resistance (R) = 6 ohms
So, the resistance of the circuit is 6 ohms.
The table on finding ohms with volts and amps
| Example | Volts (V) | Amps (A) | Ohms (Ω) |
|---|---|---|---|
| 1 | 12 | 2 | 6 |
| 2 | 24 | 1 | 24 |
| 3 | 10 | 3 | 3.33 |
| 4 | 18 | 2 | 9 |
| 5 | 15 | 2 | 7.5 |
| 6 | 30 | 1 | 30 |
| 7 | 25 | 2 | 12.5 |
| 8 | 36 | 1 | 36 |
| 9 | 20 | 3 | 6.67 |
| 10 | 48 | 1 | 48 |
| 11 | 22 | 2 | 11 |
| 12 | 54 | 1 | 54 |
| 13 | 30 | 2 | 15 |
| 14 | 60 | 1 | 60 |
| 15 | 35 | 2 | 17.5 |
| 16 | 72 | 1 | 72 |
| 17 | 40 | 2 | 20 |
| 18 | 15 | 5 | 3 |
| 19 | 80 | 1 | 80 |
| 20 | 45 | 2 | 22.5 |
| 21 | 90 | 1 | 90 |
| 22 | 50 | 2 | 25 |
| 23 | 96 | 1 | 96 |
| 24 | 55 | 2 | 27.5 |
| 25 | 100 | 1 | 100 |
| 26 | 60 | 2 | 30 |
| 27 | 110 | 1 | 110 |
| 28 | 65 | 2 | 32.5 |
| 29 | 120 | 1 | 120 |
| 30 | 70 | 2 | 35 |
How to find amps in a series circuit?
In a series circuit, the current (amps) remains the same at any given point within the circuit. Specifically, this is because there is only one path for current to flow, and the flow of electrons is continuous through all the components connected in series.
So, To find the current in a series circuit, you can follow these steps:
- Identify the known values:
- Note the voltage (V) across the power source (e.g., battery or voltage source).
- Determine the total resistance (R_total) of the entire series circuit. This can be found by adding up the individual resistances of all the components in series.
- Use Ohm’s Law:
- In a series circuit, we can write Ohm’s Law as Current (I) = Voltage (V) / Total Resistance (R_total)
- Perform the calculation:
- Divide the voltage (V) across the circuit by the total resistance (R_total) to find the current (I) flowing through the series circuit.
For example: Let’s say you have a series circuit with a voltage of 12 volts across a total resistance of 8 ohms. To find the current flowing through the circuit:
Current (I) = 12 volts / 8 ohms Current (I) = 1.5 amps
Therefore, the current flowing through the series circuit is 1.5 amperes (amps). Remember that in a series circuit, the current is the same at all points, so the calculated current value is the current flowing through any individual component within the circuit.
Note: All the content on this page and the “Watts Volts Amps Ohms Calculator” are validated by Kenan Baltaci, Bethanie Stadler and Lee Roberts. They checked the algorithm, performance and accuracy of this calculator.